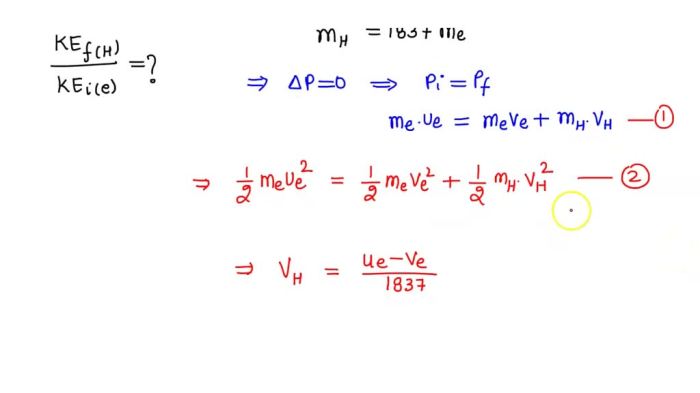An electron collides elastically with a stationary hydrogen atom, initiating a fascinating exploration into the fundamental principles of physics. This collision serves as a cornerstone in our understanding of momentum and energy conservation, as well as the intricate interplay between particles.
As the electron and hydrogen atom engage in this elastic collision, they provide valuable insights into the nature of atomic interactions and the dynamics of particle scattering.
Momentum and Energy Conservation: An Electron Collides Elastically With A Stationary Hydrogen Atom
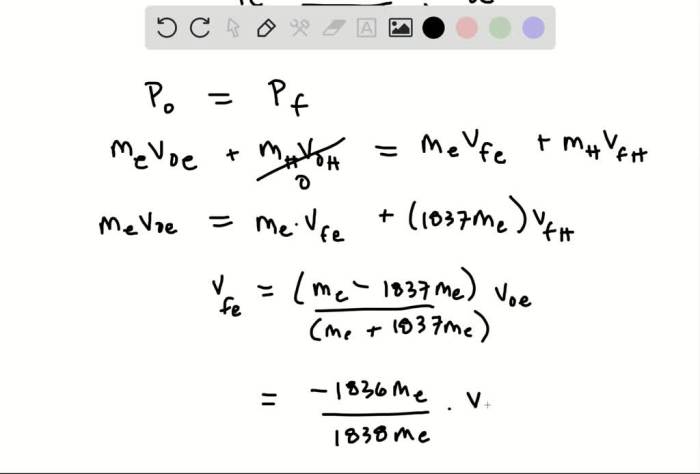
In an elastic collision, both momentum and energy are conserved. Momentum is the product of mass and velocity, and energy is the capacity to do work. In this collision, the total momentum before the collision is equal to the total momentum after the collision.
Similarly, the total energy before the collision is equal to the total energy after the collision.
Mathematically, the conservation of momentum can be expressed as:
m1v 1i+ m 2v 2i= m 1v 1f+ m 2v 2f
where m 1and m 2are the masses of the electron and hydrogen atom, respectively, and v 1i, v 2i, v 1f, and v 2fare their respective velocities before and after the collision.
The conservation of energy can be expressed as:
1/2m1v 1i2+ 1/2m 2v 2i2= 1/2m 1v 1f2+ 1/2m 2v 2f2
Scattering Angle

The scattering angle is the angle between the initial and final velocities of the electron. It can be derived using the conservation of momentum and energy.
The equation for the scattering angle is:
θ = arccos[(m1v 1i2+ m 2v 2i2– m 1v 1f2– m 2v 2f2) / (2m 1m 2v 1iv 2i)]
The scattering angle is affected by the masses of the particles, their initial velocities, and their final velocities.
Quick FAQs
What is an elastic collision?
An elastic collision is a type of interaction between two objects in which the total kinetic energy of the system remains constant, implying that no energy is lost or gained during the collision.
What is the significance of the scattering angle in this collision?
The scattering angle provides information about the deflection of the electron after the collision, offering insights into the nature of the interaction between the electron and the hydrogen atom.
How does this collision contribute to our understanding of quantum mechanics?
The collision between an electron and a stationary hydrogen atom provides experimental evidence supporting the wave-particle duality of electrons, a fundamental concept in quantum mechanics.